|
 The parabola is one of the so-called conic sections : it can be obtained as the cross section of a right circular cone when intersected by a plane parallel to one of the tangent planes to the cone. It was first studied in this way by the early Greeks. It is in fact a curve which is intermediate between an ellipse and a hperbola. If the intersecting plane is ‘flatter’ we obtain an ellipse; if it is steeper, and we think of the cone being extended to a double-cone (with an inverted cone joinng the given cone at its vertex) we obtain a hyperbola. The parabola is one of the so-called conic sections : it can be obtained as the cross section of a right circular cone when intersected by a plane parallel to one of the tangent planes to the cone. It was first studied in this way by the early Greeks. It is in fact a curve which is intermediate between an ellipse and a hperbola. If the intersecting plane is ‘flatter’ we obtain an ellipse; if it is steeper, and we think of the cone being extended to a double-cone (with an inverted cone joinng the given cone at its vertex) we obtain a hyperbola.
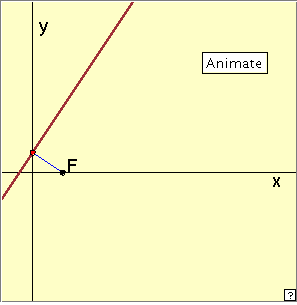
The applet below generates the parabola using a special point F called the focus, and the y-axis.
Click the diagram below to activate the applet, and then click the 'Animate' button to generate the parabola. Click the button again to stop the generation. Clicking the little red x at bottom right will clear the drawing from the applet window. Now drag the red point on d slowly up or down. Can you describe the construction?
Reflection
What is happening here? It is known that the equation of the parabola is y2 = 4ax, and that the coordinates of the focus F are (a, 0). Let the point on the y-axis be P (0, p). Then the slope of FP is –p/a, so the slope of the perpendicular to this is a/p.
Hence the perpendicular line through P has equation of the form y = ax/p + c for some constant c. The line passes through P (0, p), giving us c = p, and the equation y = ax/p + c.
From the applet, we expect this line to be tangent to the parabola.
The line meets the parabola y2 = 4ax where (ax/p + c)2 = 4ax.
Solving this for x we obtain x = p2/a, p2/a. That is, the line meets the parabola in a repeated point (coincident points) which is what we would expect for a tangent.
Occurrences
 There are many places that the parabola occurs in real life. For example, it can appear as a conic section when a flashlight shines on a wall. There are many places that the parabola occurs in real life. For example, it can appear as a conic section when a flashlight shines on a wall.
The reflective property of the parabola is used in satellite dishes, and light reflectors. In the case of the dish illustrated below left, any cross section through the vertex of the dish is parabolic in shape.
Bridges can make use of the parabolic curve in at least two different ways. Sometimes they are constructed with parabolic supporting arches (below centre). Another occurrence is with the supporting cables of a suspension bridge. The best known example of this is the Golden Gate suspension bridge in San Francisco (below right). Although a hanging cable has the shape of a catenary, where there is a load supported evenly along the length of the cable, the shape reverts to a parabola.
Another occurrence is the famous Smiley face, which in fact, has a parabolic smile!
A less easily observed occurrence of the parabola is when it occurs as the trajectory of a ball, a bullet or a rocket.
And finally there are the famous McDonald’s golden arches, which apparently are not parabolas at all! See:
|
 The parabola is one of the so-called conic sections : it can be obtained as the cross section of a right circular cone when intersected by a plane parallel to one of the tangent planes to the cone. It was first studied in this way by the early Greeks. It is in fact a curve which is intermediate between an ellipse and a hperbola. If the intersecting plane is ‘flatter’ we obtain an ellipse; if it is steeper, and we think of the cone being extended to a double-cone (with an inverted cone joinng the given cone at its vertex) we obtain a hyperbola.
The parabola is one of the so-called conic sections : it can be obtained as the cross section of a right circular cone when intersected by a plane parallel to one of the tangent planes to the cone. It was first studied in this way by the early Greeks. It is in fact a curve which is intermediate between an ellipse and a hperbola. If the intersecting plane is ‘flatter’ we obtain an ellipse; if it is steeper, and we think of the cone being extended to a double-cone (with an inverted cone joinng the given cone at its vertex) we obtain a hyperbola. 
 There are many places that the parabola occurs in real life. For example, it can appear as a conic section when a flashlight shines on a wall.
There are many places that the parabola occurs in real life. For example, it can appear as a conic section when a flashlight shines on a wall.




