FIVE OCTAHEDRA COMPOUND

    Octahedra and the icosahedron

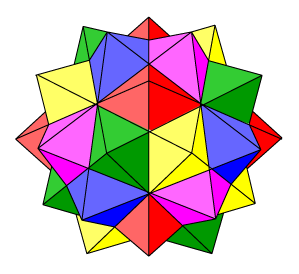
Our last compound in this section is the compound of five octahedra. Personally, I find this the least attractive of these compounds – rather like some sort of knobbly fruit! Nevertheless, it has some interesting structure. It occurs as the dual of the five cubes compound. The correspondence is:

five cubes inscribed in a dodecahedron

five octahedra intersecting in an icosahedron.

As before, we can break this model down into its five component parts.

Seeing the individual octahedra is really quite simple. Far more difficult is removing the octahedra one by one, and viewing the remains of the solid left behind. This is extraordinarily challenging to do, and I recommend this exercise to anyone wishing to improve their spatial understanding of this (and the other) compounds. So let us remove the octahedra one by one:     

  The structure and intersection of the octahedra

There are five octahedra in this compound, and each octahedron has eight faces. In the model, these faces occur in pairs in 20 planes, which enclose a regular icosahedron. A plane containing a blue triangular octahedral face and a magenta triangular octahedral face can be seen here. In the centre of the figure is an equilateral triangle which is a face of the enclosed icosahedron.

The convex hull of the five octahedra (i.e. the smallest convex set containing the octahedra) is a solid called an icosidodecahedron.



  Making the model

To make this model, check out this link. The 30 vertex pyramids are individually assembled, and then glued together. Although the resulting model is not quite rigid, it is satisfactory at normal display sizes.




  Playing with the applet

Bob has created an amazing applet here – one which allows us to understand how this compound polyhedra is constructed about a regular icosahedron.

• Play with the applet, noting that as each octahedron is deleted, an interior icosahedron is revealed.
• How many faces does the icosahedron have? How many faces of the compound contain each face of the icosahedron? How many faces of the interior icosahedron are used by each octahedron?
• Do some sums here to see why you would expect a compound of five octahedra.

Check your answers ...




  The shape of the pieces

We have already seen that each planar face of this compound contains two equilateral triangles which are faces of the octahedra.

• Remembering that the octahedron is the dual of the cube, can you explain why this happens?
(Think back to the compounds of five cubes and ten tetrahedra.)

Check your answer ...





























  The shape of the pieces

We have already seen that each planar face of this compound contains two equilateral triangles which are faces of the octahedra.

• Remembering that the octahedron is the dual of the cube, can you explain why this happens?

   In the compound of five cubes, each diagonal is shared by two cubes. The diagram at right shows the cube with vertices (1, 1, 1). Consider the vertex (1, 1, 1) with its (red) diagonal x = y = z. Constructing the dual face corresponding to this vertex, we obtain the red triangular face with edges through the midpoints A, B, C of the cube edges ending in (1, 1, 1). Notice that this red triangle is the same size as the blue triangle with vertices (–1, 1, 1), (1, –1, 1) and (1, 1, –1), and lies in a parallel plane half way between the blue triangle plane and the vertex (1, 1, 1).

Now in our work with the ten tetrahedra, we discovered that the points (0, , ––1), (––1, 0 ), (, ––1, 0) are vertices of a cube X having (1, 1, 1) as a vertex. The centroid of these three points is 1/3( – –1, – –1, – –1), which lies on the red diagonal, so cube X is the second cube with this diagonal. Further, these three new points lie in the plane of the blue triangle. (We showed this, but it is easily checked again: the plane has equation x + y + z = 2.)

Hence, in the five octahedra compound, there is another triangular face in the plane of the red triangle, and these two coplanar triangles together are congruent to the (blue) triangular coplanar faces occurring with the ten tetrahedra compound. That is, as we saw before, they are angled at c = 44° 28' (where cos c = 1/4.(3 – 2).

Hence, as above, in the face plan there are two large congruent equilateral triangles (faces of the octahedra) angled at c = 44° 28', and an enclosed equilateral triangle (a face of the enclosed icosahedron). There are six congruent scalene triangles bounding the figure. Four triangles of this shape join together to form an octahedral vertex used in making the model. Using the reflective and rotative symmetries, we see that the lengths x, y and z appear as shown. If we assume that the octahedra have edge length 1, then clearly

x + y + z = 1.

The actual values of x, y and z are given on the MathWorld site listed below. A suggested approach would be to determine one of the remaining triangle angles using the (3-dimensional) coordinates of the triangle vertices, and then use the sine rule for the triangle.

  References

MathWorld: http://mathworld.wolfram.com/Octahedron5-Compound.html

Cundy, H. M., Rollett, A. P., Mathematical Models, Oxford (2nd Edition 1961)

Wenninger, M. J., Polyhedral Models, Cambridge (1971)
  
 The icosahedron has 20 faces. Each face of the icosahedron lies in two faces of the compound. This gives a total count of 40 faces.

Since each octahedron ha eight faces, 40/8 = 5 : the number of octahedra in the compound.

    V          E         F       V – E + F  
62
180
120
2 

Obtaining these numbers is getting a little more tricky.
For V we obtain 20 + 12 for the vertices of the dodecahedron and the icosahedron, + 30 for the midpoints of one set of edges. For E counting twice the number of edges of each polyhedron gives 60 + 60, and then add 60 for three further edges on each icosahedral face. For F we obtain 120: six triangular faces for each of the 20 icosahedral faces.